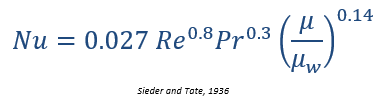This Doctor's Orders blog is a guest post from SRC Richmond's Director of Strategic Accounts, Louis Rogerson.
Low-temperature heat transfer applications regularly use anti-freezing agents like propylene glycol (PG) to enable lower-temp operation. However, at very low ambient temps, properly calculating critical fluid properties like viscosity, fluid velocity, and their impacts on heat transfer becomes more complex. This post will analyze the correlation among those properties at low ambient temperatures and provide guidance for how to best model a coil’s performance at such conditions.
The challenges of calculating PG viscosity at low ambient temps
At low temperatures – let’s say lower than ~30°F for this example – the rate of increase for PG’s viscosity (at 60% concentration) accelerates, as indicated by the curve below. For the remainder of this post, propylene glycol is used to refer to a 60% concentration of PG and water. And we're not implying that this curve holds true for other concentrations.
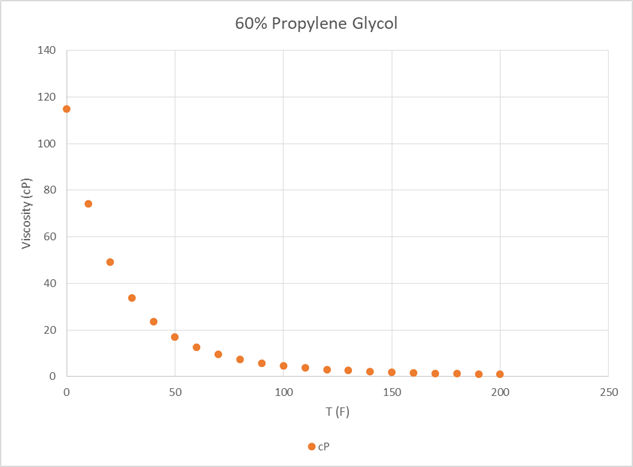
Source 1: 2017 ASHRAE handbook: fundamentals, ASHRAE, 2017
Below these temperatures, special attention is needed to properly account for variable fluid properties when modeling a fluid’s thermal performance. The use of inaccurate or incomplete values can have significant the impact on the design of the coil as well as the overall system.
Methodologies for modeling propylene glycol properties
For this portion, we’ll outline two common methodologies used to model fluid properties when designing a heat exchanger. We’ll then run those values through our coil selection software, Enterprise, to compare the results of each approach and examine their effects on coil selection.
-
Using dynamic PG property values to model its performance in the application
We consider this methodology to be the most accurate way of determining those values. It involves accounting for the nonlinear effects of a change in the ratio between the fluid’s convective and conductive heat transfer at the tube’s boundary layer. This value is represented by Nusselt number (Nu). In a heat exchanger, very low ambient temps lower the temperature of the internal tube wall, a value which varies throughout the coil.
Accurately determining the dynamic values needed for that equation involves the use of an implicit function; in this instance, the Sieder-Tate correlation (see below). Using this correlation takes into account that variability in the application’s Nusselt. The efficacy of this approach is particularly apparent in applications with very low ambient temperatures, where greater changes in the coil’s Nu exist between inlet and outlet.
Our selection software automatically accounts for variability between glycol viscosity at the heat transfer boundary surface temperature – and at the bulk viscosity temperature.
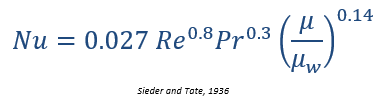
-
Using fixed PG property values to model its performance in the application
This is a common approach for propylene glycol applications featuring non-exceptional ambient temperatures. As illustrated by the graph on page one, PG’s viscosity follows a fairly predictable curve at these temperatures.
Using a constant viscosity value simplifies the correlation between the PG’s viscosity, and heat transfer coefficient by using a fixed number when a viscosity is needed for performance calcs. In environments with ambient temperatures above 30°F, the change in Nu between a coil’s inlet and outlet is unlikely to be great enough to significantly affect the calculation. But it can be unreliable in very cold ambient environments, which we illustrate below.
Rating comparison
To compare the system-wide impacts of these two performance modeling approaches, we’ve run a theoretical coil bank through our selection software, Enterprise. This coil bank consists of eight two-row coils measuring at 84” x 348” apiece and built with 5/8” tube and 6 FPI.
The working fluid was a 60% propylene glycol solution. To illustrate the impact of very low temperatures on glycol performance, our theoretical operating conditions feature -45°F ambient temps. Other conditions for the application are in the table below.

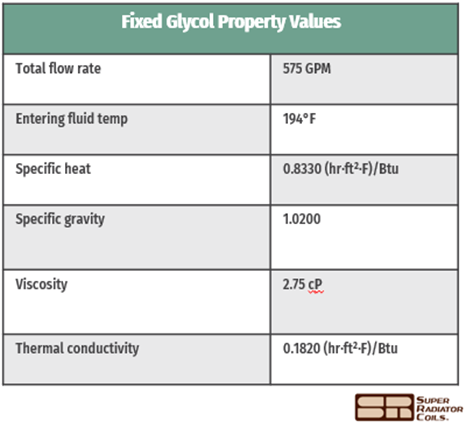
Fixed fluid property values
The table below shows the static values that will be used to calculate the PG’s performance. This method uses these predefined, average values for PG's fluid properties each time those values are needed when calculating performance ratings.
Dynamic fluid property values
For this half of the comparison, we simply swapped the static PG property values above with dynamic ones in Enterprise.
The results
The table below shows two performance models for the same coil bank – one developed using method #1 and the other using method #2.
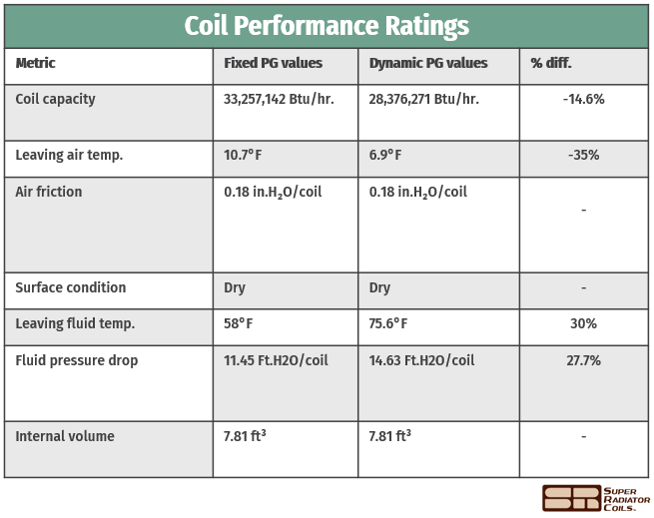
In this scenario, using fixed fluid property values for the system’s working fluid resulted in nearly a 15% performance overestimation. If this theoretical coil bank were to go into service, it wouldn’t be capable of meeting its requirement, and depending on the application, could have serious consequences.
Don’t go it alone when you’re modeling the performance of your heat exchanger. Enterprise is available for free to all our customers, and if you’re stumped or looking for some guidance, give one of expert engineers a call. We’ll work with you to understand the nuances of your application and can design your equipment to do just what you need.
Don’t get left out in the cold when it comes to heat transfer information. To stay up to date on a variety of topics on the subject, subscribe to The Super Blog, our technical blog, Doctor's Orders, and follow us on LinkedIn, Twitter, and YouTube.